how to find the coterminal angle
Ray is a licensed engineer in the Philippines. He loves to write about mathematics and civil engineering.
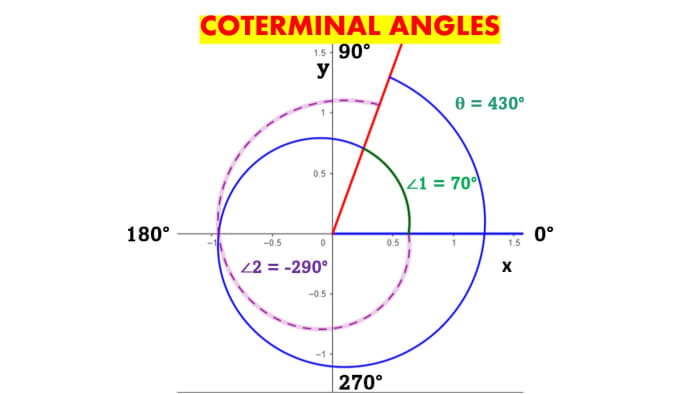
Coterminal angles are angles in a standard position that have the same terminal sides. Every angle has an unlimited number of coterminal angles. Given ∠θ in standard position with measurement xn, then the angle measures that are coterminal to the angle are given by the formula ∠θ = x° + 360°n. Take note that n is an integer. This theorem states that the measures of any two coterminal angles differ by an integer multiple of 360°. For instance, in the given figure below, θ = 430°. Shown below is a coterminal angle example that shows ∠θ and two of its coterminal angles, labeled ∠1 and ∠2.
∠1 = 430° + 360° (-1)
∠1 = 70°
∠2 = 430° + 360° (-2)
∠2 = -290°
If we add positive multiples of 360° to 430°, we can find that the angles with measures 790°, 1150°, 1510°, are also coterminal with the given θ = 430°.
Coterminal Angles
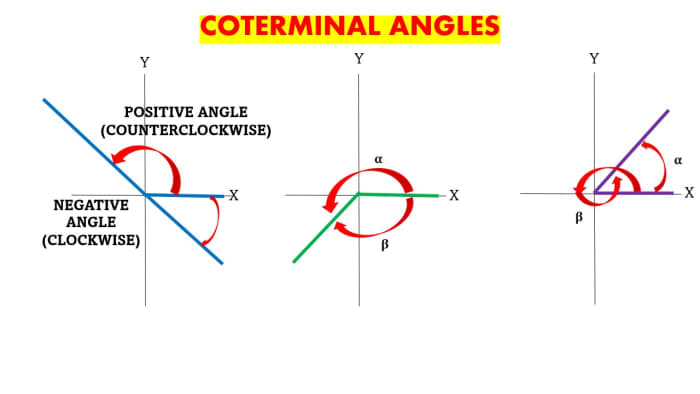
Write down these few notes to be able to learn thoroughly about coterminal angles. Rotate a ray or a half-line about its endpoint to determine an angle. The starting position of the ray is the initial side of the angle, and the position after rotation is the terminal side. The origin is the vertex in the coordinate system, and the initial side coincides with the positive x-axis; such an angle is in a standard position. Another thing to remember is that counterclockwise rotation generates positive angles, and clockwise rotation generates negative angles. Lastly, always remember that two angles are coterminal if the difference of their degree measures is evenly divisible by 360°.
Coterminal Angles in Clockwise and Counterclockwise Directions
Let us see the coterminal angles of some basic trigonometric angles shown in the question and answer table.
| Frequently Asked Questions (FAQ) | Answers |
|---|---|
| What is the coterminal angle of 30°? | Some of the coterminal angles of 30° are 390°, 750°, -330°, and -690°. The formula ∠θ = 30° + 360°n represents the coterminal angles of 30°, where n is an integer. |
| What is the coterminal angle of 45°? | Few of the coterminal angles of 45° are 405°, 765°, -315°, and -675°. The formula ∠θ = 45° + 360°n represents the coterminal angles of 45°, where n is an integer. |
| What is the coterminal angle of 60°? | Some of the coterminal angles of 60° are 420°, 780°, -300°, and -660°. The formula ∠θ = 60° + 360°n represents the coterminal angles of 60°, where n is an integer. |
| What is the coterminal angle of 90°? | The coterminal angles of 90° are 450°, 810°, -270°, and -630°. |
| What is the coterminal angle of 120°? | The coterminal angles of 120° are 480°, 840°, -240°, and -600°. |
| What is the coterminal angle of 112°? | Some of the coterminal angles of 112° are 472°, 832°, -248°, and -608°. |
| What is the coterminal angle of 220°? | Some of the coterminal angles of 220° are 580°, 940°, -140°, and -500°. |
How to Find Coterminal Angles in Degrees
In finding coterminal angles, note that their measures must differ by an integer multiple of 360°.
- Case 1: Given Positive Angle and Greater than 360°. To find the smallest positive coterminal angles, subtract 360° repeatedly until the result is a positive angle less than or equal to 360°.
- Case 2: Given a Non-Positive Angle. In finding coterminal angles of a non-positive angle, add 360° repeatedly until the result is a positive angle less than or equal to 360°.
How to Find Coterminal Angles in Radians
Two angles are coterminal when they have the same initial and terminal sides, and another way to measure angles is in radians. One radian is the measure of a central angle θ that intercepts an arc s equal in length to the radius r of the circle. It means that θ = s / r where θ is in radians. Since the circumference of a circle is 2
How to Find Coterminal Angles in Radians
The same principle goes with finding coterminal angles in radians. For instance, angles 0 and 2
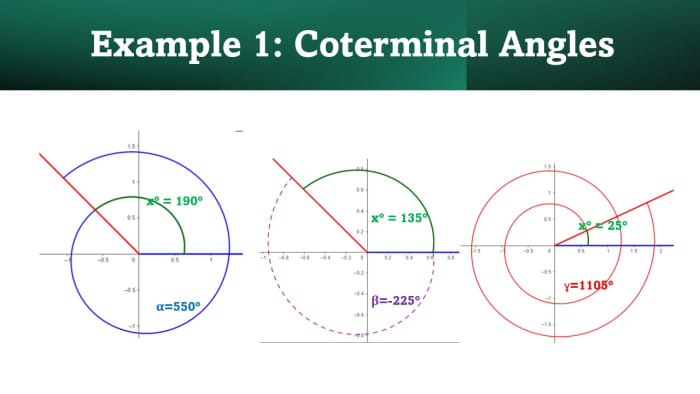
Example 1: Finding Coterminal Angles and Classifying by Quadrant
Assume that the given angles are in standard position. Determine the measure of the positive angle with a measure less than 360° that is coterminal with the given angle. Then, classify the angle by quadrant.
- ɑ = 550°
- β = -225°
- ɣ = 1105°
Solution
Start the solution by writing the formula for coterminal angles. Let ∠θ = ∠ɑ = ∠β = ∠ɣ. Solve for the angle measure of x° for each of the given angles in standard position. The resulting solution, ∠ɑ, is a Quadrant III angle while the ∠β is a Quadrant II angle.
∠θ = x° + 360°n
∠ɑ = x° + 360°(1)
550° = x° + 360°
x° = 550° - 360°
x° = 190°
∠θ = x° + 360°n
∠β = x° + 360°n
-225° = x° + 360°(-1)
x° = -225° + 360°
x° = 135°
Since ɣ = 1105° exceeds the single rotation in a cartesian plane, we must know the standard position angle measure. The solution below, ∠ɣ, is an angle formed by three complete counterclockwise rotations, plus 5/72 of a rotation. In converting 5/72 of a rotation to degrees, multiply 5/72 with 360°.
ɣ = 1105° / 360°
ɣ = 3- 5/72
(5//72) x (360°) = 25°
Therefore, incorporating the results to the general formula:
∠θ = x° + 360°n
∠ɣ = x° + 360°(n)
1105° = x° + 360°(3)
x° = 1105° - 1080°
x° = 25°
Final Answer
Therefore, the positive coterminal angles (less than 360°) of ∠ɑ = 550°, β = -225°, and ɣ = 1105° is 190°, 135°, and 25°, respectively.
Finding Coterminal Angles and Classifying by Quadrant
Example 2: Coterminal Angles Example
Determine whether the following pairs of angles are coterminal.
- ɑ = 120°, β = -180°
- ɑ = 20°, β = 740°
Solution
In determining if ɑ = 120° is coterminal with β = -180°, plot the angle measures in a quadrant system. As observed from the plot, the terminal side of ɑ is in quadrant II, while the terminal side of β is along the negative x-axis. Therefore, angles ɑ and β are not coterminal angles.
For letter b, before we proceed with plotting, note that β = 740° represents two rotations of 360°. Therefore, after 720° of rotation, the angle is again along the positive x-axis. To make it 740°, add another 20° of rotation in the positive direction to achieve a 740° angle. With that, β = 740° lies in the first quadrant (QI).
Final Answer
Since ɑ = 120°, β = -180° have different coterminal side, they are not coterminal angles.
Since ɑ = 20°, β = 740° have the same terminal side; then they are coterminal angles.
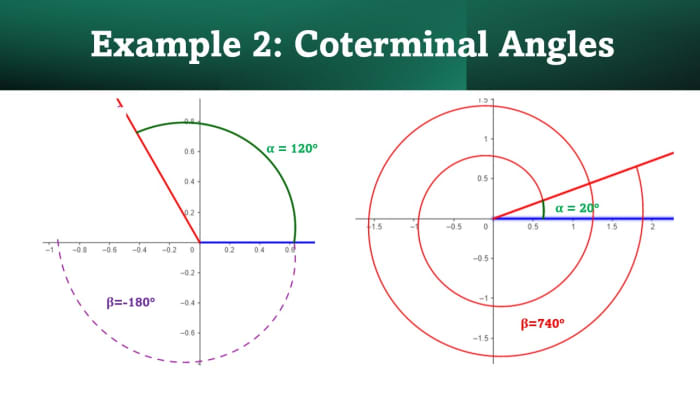
Coterminal Angles Example
Example 3: Finding Coterminal Angles
Determine the angle of the smallest positive measure that is coterminal with each of the following angles.
- 820°
- -520°
Solution
The given angle measure in letter a is positive. Since it is a positive angle and greater than 360°, subtract 360° repeatedly until one obtains the smallest positive measure that is coterminal with measure 820°.
820° - 360° = 460°
460° - 360° = 100°
Take note that -520° is a negative coterminal angle. Since the given angle measure is negative or non-positive, add 360° repeatedly until one obtains the smallest positive measure of coterminal with the angle of measure -520°.
-520° + 360° = -160°
-160° + 360° = 200°
Final Answer
Therefore, the angles with measure 100° and 200° are the angles with the smallest positive measure that is coterminal with the angles of measure 820° and -520°, respectively.
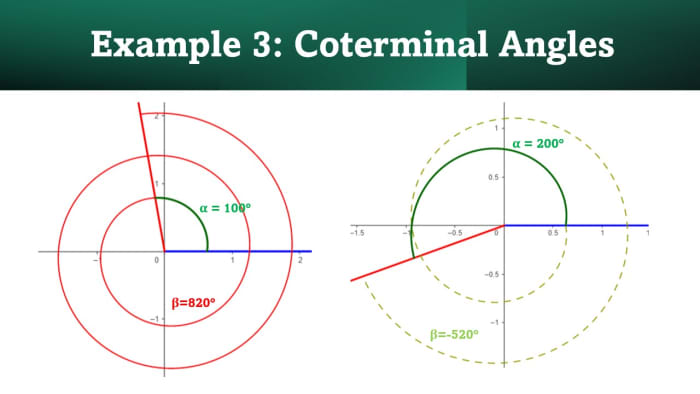
Finding Coterminal Angles
Example 4: How to Find Coterminal Angles in Radians
For the positive angle 13
Solution
For the positive angle 13
Final Answer
Therefore the coterminal angles of 13
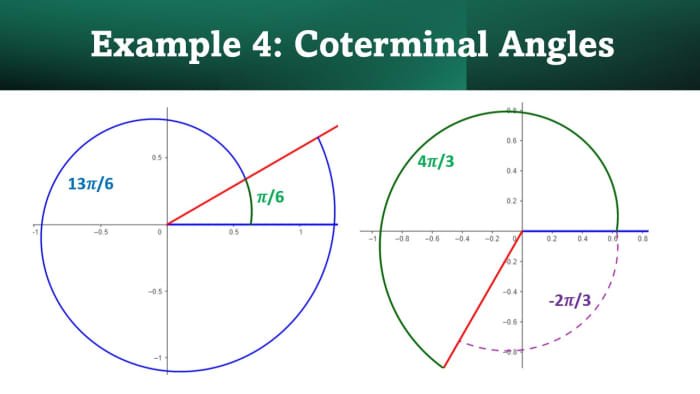
How to Find Coterminal Angles in Radians
Example 5: Finding All Coterminal Angles
Find all angles that are coterminal with 120°.
Solution
For any integer k, 120° + 360° k will be coterminal with 120°. Shown below are some of the coterminal angles of 120°.
At k = -2
∠θ = 120° + 360° (-2)
∠θ = 120° - 720°
∠θ = -600°
At k = -1
∠θ = 120° + 360° (-1)
∠θ = 120° - 360°
∠θ = -240°
At k = 1,
∠θ = 120° + 360° (1)
∠θ = 480°
At k = 2.
∠θ = 120° + 360° (2)
∠θ = 120° + 720°
∠θ = 840°
Final Answer
Therefore, the formula ∠θ = 120° + 360° k represents the coterminal angles of 120°.
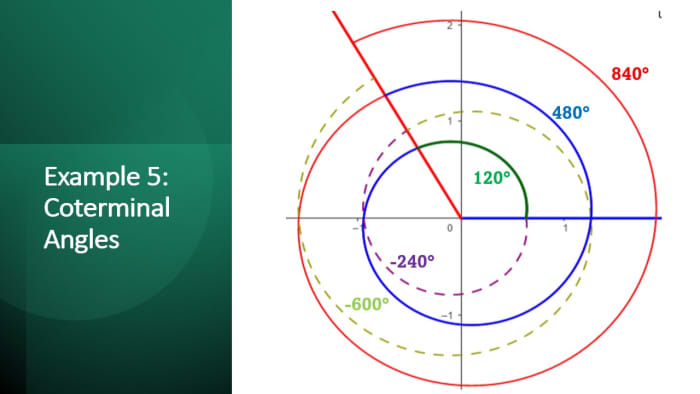
Finding All Coterminal Angles of 120 Degrees
Example 6: Determining Two Coterminal Angles and Plotting
Draw - 90° in standard position. Then determine two coterminal angles, two positive and two negatives, that are coterminal with -90°.
Solution
The figure below shows -90° in standard position. In determining positive and negative coterminal angles, traverse a full revolution in the positive and negative directions on the coordinate system. Let x° = -90°.
∠θ = x° + 360° (k)
∠θ = -90° + 360° (1)
∠θ = 270°
∠θ = x° + 360° (k)
∠θ = -90° + 360° (2)
∠θ = 630°
∠θ = x° + 360° (k)
∠θ = -90° + 360° (-1)
∠θ = -450°
∠θ = x° + 360° (k)
∠θ = -90° + 360° (-2)
∠θ = -810°
Final Answer
Therefore, 270° and 630° are two positive angles coterminal with -90°. On the other hand, -450° and -810° are two negative angles coterminal with -90°.
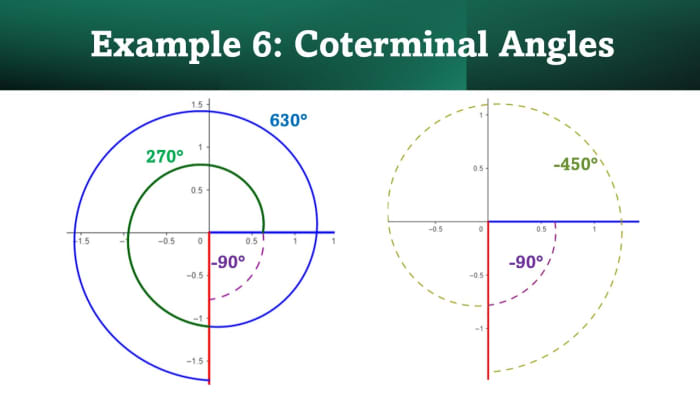
Determining Two Coterminal Angles and Plotting
Example 7: Coterminal Angle Theorem and Reference Angle Theorem
Find the exact value of cos (495°).
Solution
Let us find the coterminal angle by subtracting 360° from the given angle 495°. Now, reference angles, sometimes called related angles, are positive acute angles between the terminal side of θ and the x-axis for any angle θ in standard position. The reference angle for 135° is 45°. Since 495° terminates in quadrant II, its cosine is negative.
495° - 360° = 135°
cos (495°) = cos (135°)
cos (495°) = - cos (45°)
cos (495°) = - √2/2
Final Answer
The exact value of cos (495°) is - √2/2.
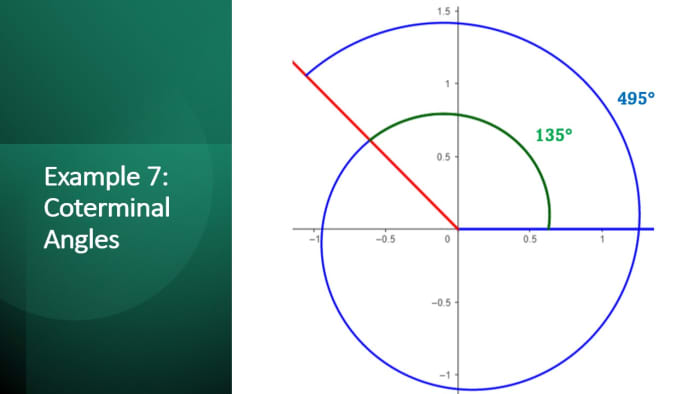
Coterminal Angle Theorem and Reference Angle Theorem
Example 8: Finding Measures of Coterminal Angles
Find the angles of least positive measure that are coterminal with each angle.
- 908°
- -75°
- -440°
Solution
For the given angle measure of 908°, subtract 360° multiple times as needed to obtain an angle with a measure greater than 0° but less than 360°.
908° - 360° = 548°
620° - 360° = 188°
For letter b with the given angle measure of -75°, add 360°.
-75° + 360° = 285°
Lastly, for letter c with an angle measure of -440°, add 360° multiple times to achieve the least positive coterminal angle.
-440° + 360° = -80°
-80° + 360° = 280°
Final Answer
The positive angle measures coterminal with 908°, -75°, and -440° are 188°, 285°, and 280°, respectively.
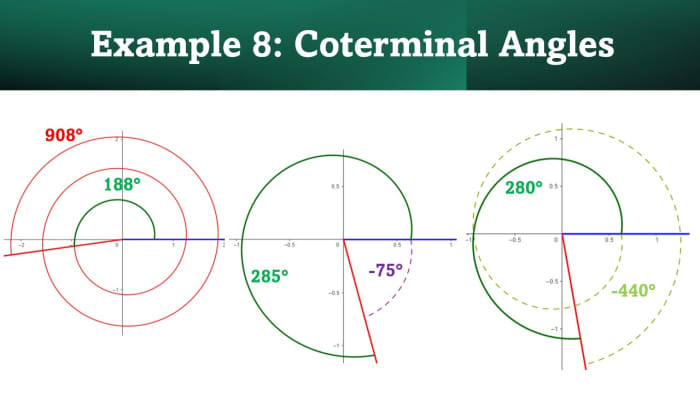
Finding Measures of Coterminal Angles
Example 9: Finding Coterminal Angles and Reference Angles
Let θ = 928°, then find the following:
- Which angle between 0° and 360° has the same terminal side as θ?
- What is the reference angle for θ?
Solution
Reduce the given θ to the smallest possible coterminal angle between 0° and 360° by adding 360° several times if needed.
928° - 360° = 568°
568° - 360° = 208°
Let us identify the quadrant the coterminal angles reside. Since 928° and 208° have the same terminal side in quadrant III, so the reference angle for θ = 928° is identifiable by subtracting 180° from the coterminal angle between 0° and 360°.
208° - 180° = 28°
Final Answer
The angle between 0° and 360° has the same terminal side as θ = 928° is 208° and the reference angle is 28°.
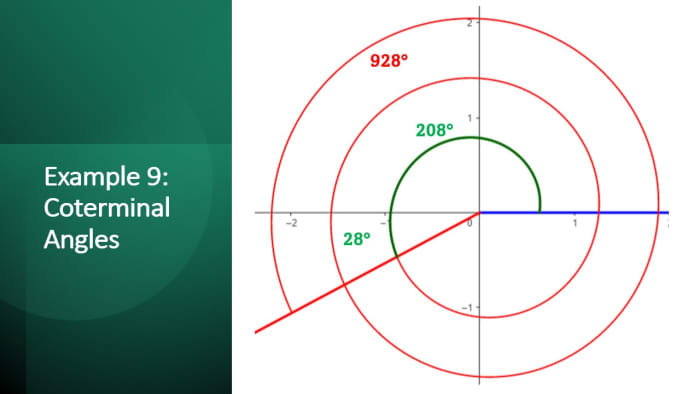
Finding Coterminal Angles and Reference Angles
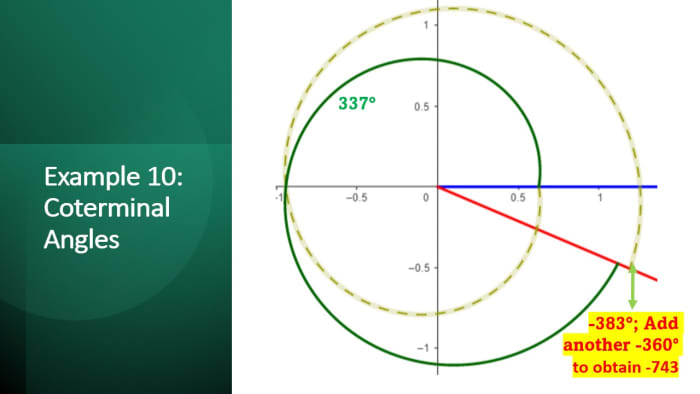
Example 10: Coterminal Primary Angles
What is the primary angle coterminal with the angle of -743°?
Solution
Write the equation following the general formula for coterminal angles ∠θ = x° + 360°n given that ∠θ = -743.
-743° = x° - 360°n
-743° = x° - 360°(3)
-743° = x° - 1080°
Multiply both sides of the equation by -1. Note to choose the positive integer value for n results in an angle that is larger than but closest to the angle of 743°.
-1 (-743°) = -1 (x° - 1080°)
743° = -x° + 1080°
x° = 1080° - 743°
x° = 337°
Final Answer
The primary angle coterminal with ∠θ = -743 is x° = 337°.
Coterminal Primary Angles
This content is accurate and true to the best of the author's knowledge and is not meant to substitute for formal and individualized advice from a qualified professional.
© 2021 Ray
how to find the coterminal angle
Source: https://owlcation.com/stem/Coterminal-Angles
Posted by: goodsoncined1957.blogspot.com

0 Response to "how to find the coterminal angle"
Post a Comment